Solved Problem on Two-dimensional Motion
advertisement
Three identical spheres are launched from the same height H with the same speeds. Sphere A
is thrown vertically downward, B is thrown vertically upward, and C is thrown horizontally.
Which one gets to the ground at a higher speed, neglecting the resistance of the air.

Problem data:
- Initial speed: v0;
- Height of the release point: h.
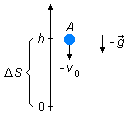
- Sphere A:
\[
\begin {gather}
\bbox[#99CCFF,10px]
{v^2=v_0^2+2a\Delta S} \tag{I}
\end{gather}
\]
\[
\begin{gather}
v^2=(-v_0)^2+2(-g)\Delta S\\[5pt]
v^2=v_0^2-2g(0-h)\\[5pt]
v^2=v_0^2-2g(-h)\\[5pt]
v^2=v_0^2+2gh\\[5pt]
v=\sqrt{v_0^2+2gh} \tag{II}
\end{gather}
\]
- Sphere B:
In the first part, the initial speed of the launch is positive, it is oriented in the same direction as the reference frame, and the final speed is zero, vH = 0, which is the instant the sphere stops and reverses the motion to begin to fall (Figure 2-A).
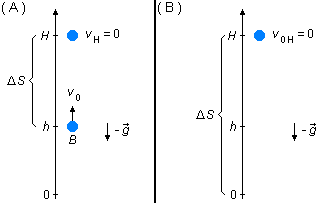
Writing the expression (I) for the first part, we find the height hit by the sphere
\[
\begin{gather}
v^2=v_0^2+2a\Delta S\\[5pt]
v_H^2=v_0^2+2(-g)\Delta S\\[5pt]
0^2=v_0^2-2g(H-h)\\[5pt]
0=v_0^2-2gH+2gh\\[5pt]
2gH=v_0^2+2gh\\[5pt]
H=\frac{v_0^2+2gh}{2g} \tag{III}
\end{gather}
\]
In the second part, free fall, the initial speed will be the final speed of the first part, equal
to zero, v0H = vH = 0, and the initial height will be given by
the expression (III). Applying the expression (I), we have the speed with which the sphere reaches the
ground
\[
\begin{gather}
v^2=v_0^2+2a\Delta S\\[5pt]
v^2=v_{0H}^2+2(-g)\Delta S\\[5pt]
v^2=0^2-2g(0-H)\\[5pt]
v^2=0-2g(-H)\\[5pt]
v^2=0+2gH\\[5pt]
v^2=\cancel{2g}\left(\frac{v_0^2+2gh}{\cancel{2g}}\right)\\[5pt]
v=\sqrt{v_0^2+2gh} \tag{IV}
\end{gather}
\]
- Sphere C:
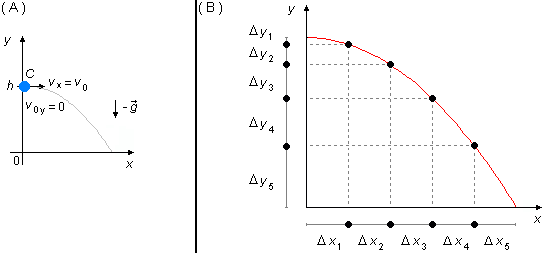
In the x direction, there is no acceleration acting on the sphere, it moves uniformly, for each time interval the displacements along the x-axis are the same (Δ x1=Δ x2=Δ x3=Δ x4=Δ x5 - Figura 3-B). The component of speed in that direction will be the speed of launching the sphere v0
\[
\begin{gather}
v_x=v_0 \tag{V}
\end{gather}
\]
In the y direction, we have the acceleration due to gravity acting on the sphere, for the same time
interval the displacements are increasing (Δ y1<Δ
y2<Δ y3<Δ y4<Δ
y5). Initially, the speed is zero v0y = 0, the speed in that direction
is given by the expression (I), with a = −g
\[
\begin{gather}
v_y^2=v_{0y}^2+2(-g)\Delta S_y\\[5pt]
v_y^2=0^2-2g(0-h)\\[5pt]
v_y^2=0-2g(-h)\\[5pt]
v_y^2=2gh \tag{VI}
\end{gather}
\]
The speed with which the sphere reaches the ground is given by the sum of the components in the x
and y directions given by the expressions (V) and (VI) respectively, using the
Pythagorean Theorem (Figure 4)
\[
\begin{gather}
v^2=v_{x}^2+v_y^2\\[5pt]
v^2=v_0^2+2gh\\[5pt]
v=\sqrt{v_0^2+2gh} \tag{VII}
\end{gather}
\]
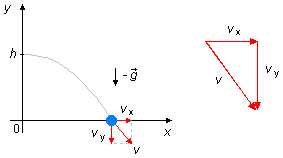
Comparing expressions (II), (IV), and (VII), we see that all spheres come to the ground at the same speed.
advertisement

Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .