Solved Problem on One-dimensional Motion
advertisement
The motion of a car, which moves with constant speed, is described by the following table:
| t (h) | 1 | 2 | 4 | 7 | 9 | 11 | 12 |
|---|---|---|---|---|---|---|---|
| S (km) | 100 | 200 | 450 | 600 | 400 | 200 | 100 |
from the table data find:
a) The average speed of the car between the instants 1 h and 2 h;
b) The average speed of the car between the instants 4 h and 7 h;
c) The average speed of the car between the instants 9 h and 12 h;
d) The average speed of the car between the instants 1 and 12 h.
Problem diagram:
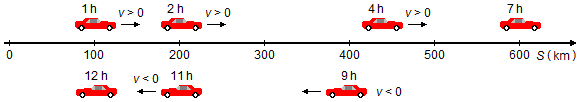
We choose a reference frame oriented to the right.
Solution
a) Using the equation for the average speed
\[
\begin{gather}
\bbox[#99CCFF,10px]
{\bar v=\frac{\Delta S}{\Delta t}=\frac{S_f-S_i}{t_f-t_i}} \tag{I}
\end{gather}
\]
\[
\begin{gather}
\bar v=\frac{200\;\mathrm{km}-100\;\mathrm{km}}{2\;\mathrm h-1\;\mathrm h}\\[5pt]
\bar v=\frac{100\;\mathrm{km}}{1\;\mathrm h}
\end{gather}
\]
\[
\begin{gather}
\bbox[#FFCCCC,10px]
{v_m=100\;\mathrm{km/h}}
\end{gather}
\]
b) Using the equation (I) for the average speed
\[
\begin{gather}
\bar v=\frac{600\;\mathrm{km}-450\;\mathrm{km}}{7\;\mathrm h-4\;\mathrm h}\\[5pt]
\bar v=\frac{150\;\mathrm{km}}{3\;\mathrm h}
\end{gather}
\]
\[
\begin{gather}
\bbox[#FFCCCC,10px]
{\bar v=50\;\mathrm{km/h}}
\end{gather}
\]
c) Using the equation (I) for the average speed
\[
\begin{gather}
\bar v=\frac{100\;\mathrm{km}-400\;\mathrm{km}}{12\;\mathrm h-9\;\mathrm h}\\[5pt]
\bar v=-\frac{300\;\mathrm{km}}{3\;\mathrm h}
\end{gather}
\]
\[
\begin{gather}
\bbox[#FFCCCC,10px]
{\bar v=-100\;\text{km/h}}
\end{gather}
\]
d) Using the equation (I) for the average speed
\[
\begin{gather}
\bar v=\frac{100\;\mathrm{km}-100\;\mathrm{km}}{12\;\mathrm h-1\;\mathrm h}\\[5pt]
\bar v=\frac{0}{11\;\mathrm h}
\end{gather}
\]
\[
\begin{gather}
\bbox[#FFCCCC,10px]
{\bar v=0}
\end{gather}
\]
Note: The average zero does not mean that the car remained at rest. If the average speed is
zero, the object may remain at rest in the same position all the time or may have moved and returned to
the same position (as happened here). The average speed gives an overview of the change of speed but does not
indicate the point variations that occurred during the movement.
advertisement

Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .