Solved Problem on Harmonic Oscillations
advertisement
A block of mass m = 2.50 kg is attached to a spring with a spring constant k = 12.00 N/m
and a shock absorber with a damping coefficient b = 0.60 N.s/m. The block is displaced from its
equilibrium position O to a point x0 at 0.20 m and released from rest.
Determine:
a) The equation of motion;
b) What is the type of oscillator?
c) The graph of position x versus time t.
a) The equation of motion;
b) What is the type of oscillator?
c) The graph of position x versus time t.

Problem data:
- Mass of the body: m = 2.50 kg;
- Spring constant: k = 12.00 N/m;
- Damping coeficient: b = 0.60 N.s/m;
- Initial position (t = 0): x0 = 0.20 m;
- Initial velocity (t = 0): v0 = 0.
We choose a reference frame with a positive direction pointing to the right. The block is moved to the
position x0 = 0.20 m and released from rest, v0 = 0. When released,
the spring force will act toward the equilibrium position (Figure 1). With this, we write the
Initial Conditions of the problem
\[
\begin{gather}
x(0)=0.20\;\text{m}\\[10pt]
v_{0}=\frac{dx}{dt}=0
\end{gather}
\]
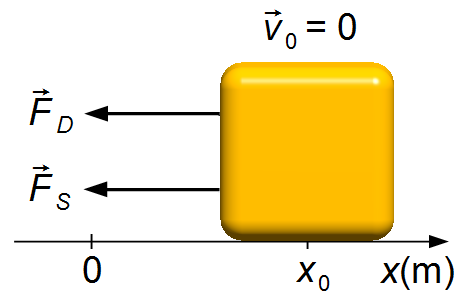
Solution
a) Applying Newton's Second Law (Figure 1)
\[
\begin{gather}
\bbox[#99CCFF,10px]
{F=m\frac{d^{2}x}{dt^{2}}} \tag{I}
\end{gather}
\]
the forces acting on the block are the spring force
\( {\vec{F}}_{S} \)
and the damping force
\( {\vec{F}}_{D} \)
given, in magnitude, by
\[
\begin{gather}
\bbox[#99CCFF,10px]
{F_{S}=-kx} \tag{II-a}
\end{gather}
\]
\[
\begin{gather}
\bbox[#99CCFF,10px]
{F_{D}=-bv=-b\frac{dx}{dt}} \tag{II-b}
\end{gather}
\]
the minus sign in the spring force means that it acts against the direction of displacement of the block
(acts toward the equilibrium), in the damping force means that it acts against the direction of the velocity
(acts in the direction of braking the movement). Substituting the expressions of (II) into expression (I)
\[
\begin{gather}
-kx-b\frac{dx}{dt}=m\frac{d^{2}x}{dt^{2}}\\[5pt]
m\frac{d^{2}x}{dt^{2}}+b\frac{dx}{dt}+kx=0
\end{gather}
\]
this is a Second Order Homogeneous Differential Equation. Dividing the equation by the mass m
\[
\begin{gather}
\frac{d^{2}x}{dt^{2}}+\frac{b}{m}\frac{dx}{dt}+\frac{k}{m}x=0
\end{gather}
\]
substituting the values given in the problem
\[
\begin{gather}
\frac{d^{2}x}{dt^{2}}+\frac{0.60}{2.50}\frac{dx}{dt}+\frac{12.00}{2.50}x=0\\[5pt]
\frac{d^{2}x}{dt^{2}}+0.24\frac{dx}{dt}+4.80x=0 \tag{III}
\end{gather}
\]
Solution of \( \frac{d^{2}x}{dt^{2}}+0.24\frac{dx}{dt}+4.80x=0 \)
The solution to this type of equation is found substituting
The solution to this type of equation is found substituting
\[
\begin{gather}
x=\operatorname{e}^{\lambda t}\\[5pt]
\frac{dx}{dt}=\lambda \operatorname{e}^{\lambda t}\\[5pt]
\frac{d^{2}x}{dt^{2}}=\lambda^{2}\operatorname{e}^{\lambda t}
\end{gather}
\]
substituting these values into the differential equation
\[
\begin{gather}
\lambda^{2}\operatorname{e}^{\lambda t}+0.24\lambda\operatorname{e}^{\lambda t}+4.80\operatorname{e}^{\lambda t}=0\\[5pt]
\operatorname{e}^{\lambda t}\left(\lambda ^{2}+0.24\lambda+4.80\right)=0\\[5pt]
\lambda^{2}+0.24\lambda+4.80=\frac{0}{{\operatorname{e}}^{\lambda t}}\\[5pt]
\lambda^{2}+0.24\lambda +4.80=0
\end{gather}
\]
this is the Characteristic Equation that has a solution
\[
\begin{gather}
\Delta=b^{2}-4ac=0.24^{2}-4\times 1\times 4.80=0.06-19.20=-19.14
\end{gather}
\]
for Δ<0, the roots are complex of the form a+bi, where
\( i=\sqrt{-1} \)
\[
\begin{gather}
\lambda=\frac{-b\pm \sqrt{\Delta\;}}{2a}=\frac{-0.24\pm \sqrt{-19.14\;}}{2\times 1}=\frac{-0.24\pm4.37i}{2}\\[5pt]
\lambda_{1}=-0.12+2.19i\qquad \text{e}\qquad \lambda_{2}=-0.12-2.19i
\end{gather}
\]
the solution of the differential equation will be
\[
\begin{gather}
x=C_{1}\operatorname{e}^{\lambda_{1}t}+C_{2}\operatorname{e}^{\lambda_{2}t}\\[5pt]
x=C_{1}\operatorname{e}^{(-0.12+2.19i)t}+C_{2}\operatorname{e}^{(-0.12-2.19i)t}\\[5pt]
x=C_{1}\operatorname{e}^{(-0.12t+2.19it)}+C_{2}\operatorname{e}^{(-0.12t-2.19it)}\\[5pt]
x=C_{1}\operatorname{e}^{-0.12t}\operatorname{e}^{2.19it}+C_{2}\operatorname{e}^{-0.12t}\operatorname{e}^{-2.19it}\\[5pt]
x=\operatorname{e}^{-0.12t}\left(C_{1}\operatorname{e}^{2.19it}+C_{2}\operatorname{e}^{-2.19it}\right)
\end{gather}
\]
where C1 and C2 are constants of integration, using
Euler's Formula
\( \operatorname{e}^{i\theta }=\cos \theta +i\sin \theta \)
\[
\begin{gather}
x=\operatorname{e}^{-0.12t}\left[C_{1}\left(\cos2.19t+i\sin 2.19t\right)+C_{2}\left(\cos2.19t-i\sin 2.19 t\right)\right]\\[5pt]
x=\operatorname{e}^{-0.12t}\left[C_{1}\cos2.19t+iC_{1}\sin 2.19t+C_{2}\cos2.19t-iC_{2}\sin 2.19t\right]\\[5pt]
x=\operatorname{e}^{-0.12t}\left[\left(C_{1}+C_{2}\right)\cos2.19t+i\left(C_{1}-C_{2}\right)\sin 2.19t\right]
\end{gather}
\]
defining two new constants α and β in terms of C1 e
C2
\[
\begin{gather}
\alpha \equiv C_{1}+C_{2}\\[5pt]
\text{e}\\[5pt]
\beta \equiv i(C_{1}-C_{2})
\end{gather}
\]
\[
\begin{gather}
x=\operatorname{e}^{-0.12t}\left(\alpha \cos 2.19t+\beta\sin 2.19t\right) \tag{IV}
\end{gather}
\]
Differentiation of
\[
\begin{gather}
x=\underbrace{\operatorname{e}^{-0.12t}}_{u}\underbrace{\left(\alpha\cos 2.19t+\beta \sin 2.19t\right)}_{v}
\end{gather}
\]
usding the Product Rule for differentiation of functions
\[
\begin{gather}
(uv)'=u'v+uv'
\end{gather}
\]
where
\( u=\operatorname{e}^{-0.12t} \)
and
\( v=\left(\alpha \cos 2.19t+\beta \sin 2.19t\right) \),
the term in parentheses is the derivative of the sum given by the sum of derivatives
\[
\begin{gather}
(f+g)'=f'+g'
\end{gather}
\]
and the sine and cosine functions in parentheses are composite functions whose derivatives are given by
the Chain Rule
\[
\begin{gather}
\frac{df[w(t)]}{dt}=\frac{df}{dw}\frac{dw}{dt}
\end{gather}
\]
with
\( f=\alpha \cos w \),
\( g=\beta \sin w \)
and
\( w=2.19t \)
\[
\begin{gather}
\frac{dx}{dt} =\frac{du}{dt}v+u\frac{dv}{dt}\\[5pt]
\frac{dx}{dt} =\frac{du}{dt}v+u\left(\frac{df}{dt}+\frac{dg}{dt}\right)\\[5pt]
\frac{dx}{dt} =\frac{du}{dt}v+u\left(\frac{df}{dw}\frac{dw}{dt}+\frac{dg}{dw}\frac{dw}{dt}\right)\\[5pt]
\frac{dx}{dt} =\frac{d\left(\operatorname{e}^{-0.12t}\right)}{dt}\left(\alpha\cos 2.19t+\beta\sin 2.19t\right)+\\
+\left(\operatorname{e}^{-0.12t}\right)\left[\frac{d(\alpha\cos w)}{dw}\frac{d(2.19t)}{dt}+\frac{d(\beta\sin w)}{dw}\frac{d(2.19t)}{dt}\right]\\[5pt]
\frac{dx}{dt} =-0.12\operatorname{e}^{-0.12t}\left(\alpha\cos 2.19t+\beta\sin 2.19t\right)+\\
+\left(\operatorname{e}^{-0.12t}\right)\left[(-\alpha\sin w)(2.19)+(\beta \cos w)(2.19)\right]\\[5pt]
\frac{dx}{dt} =\operatorname{e}^{-0.12t}\left[\left(-0.12\alpha\cos 2.19t-0.12\beta\sin 2.19t\right)\right.+\\
+\left.\left(-2.19\alpha\sin 2.19t+2.19\beta \cos2.19t\right)\right]\\[5pt]
\frac{dx}{dt} =\operatorname{e}^{-0.12t}\left[\left(-0.12\alpha\cos 2.19t-2.19\alpha\sin 2.19t\right)\right.+\\
+\left.\left(-0.12\beta\sin 2.19t+2.19\beta \cos2.19t\right)\right]\\[5pt]
\frac{dx}{dt} =\operatorname{e}^{-0.12t}\left[-\alpha\left(0.12\cos 2.19t+2.19\sin 2.19t\right)\right.+\\
+\left.\beta\left(2.19\cos2.19t-0.12\sin 2.19t\right)\right] \tag{V}
\end{gather}
\]
Substituting the Initial Conditions in the expressions (IV) and (V)
\[
\begin{gather}
x(0)=0.20=\operatorname{e}^{-0.12\times 0}\left(\alpha \cos 0.12\times 0+\beta \sin 0.12\times 0\right)\\[5pt]
\alpha=0.20 \tag{VI}
\end{gather}
\]
\[
\begin{gather}
\frac{dx(0)}{dt}=0=\operatorname{e}^{-0.12\times 0}\left[-0.20\times \left(0.12\cos2.19\times 0+2.19\sin 2.19\times 0\right)\right.\text{+}\\
\text{+}\left.\beta\left(2.19\cos2.19\times 0-0.12\sin 2.19\times 0\right)\right]\qquad\\[5pt]
0=-0.20\times \left(0.12\times 1+0\right)+\beta\left(2.19\times 1-0\right)\\[5pt]
0.02=2.19\beta \\[5pt]
\beta=\frac{0.02}{2.19}\\[5pt]
\beta =0.01 \tag{VII}
\end{gather}
\]
substituting the constants (VI) and (VII) in the expression (IV)
\[
\begin{gather}
x=\operatorname{e}^{-0.12t}\left(0.20\cos2.19t+0.01\sin 2.19t\right)
\end{gather}
\]
Equation of motion
\[
\begin{gather}
\bbox[#FFCCCC,10px]
{x(t)=\operatorname{e}^{-0.12t}\left(0.20\cos2.19t+0.01\sin 2.19t\right)}
\end{gather}
\]
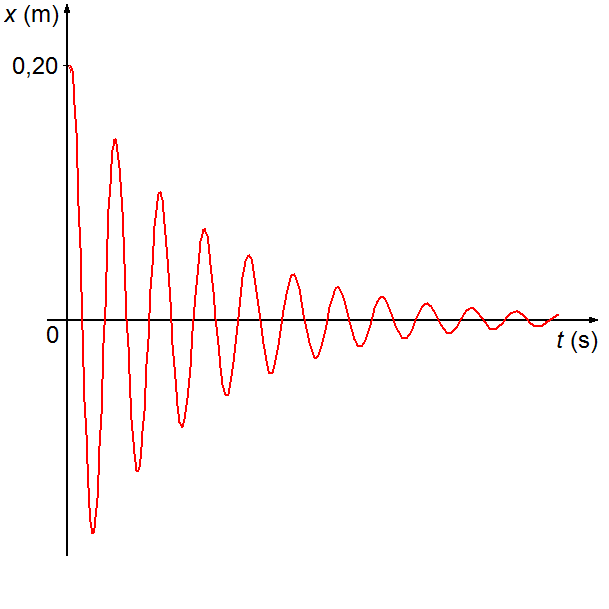
b) As Δ<0 this is an underdamped oscillator.
c) Plotting the graph of
\[
\begin{gather}
x(t)=\operatorname{e}^{-0.12t}\left(0.20\cos2.19t+0.01\sin 2.19t\right)
\end{gather}
\]
The function x(t) is the product of two functions,
\( f(t)=\operatorname{e}^{-0.12t} \)
and
\( g(t)=0.20\cos 2.19t+0.01\sin 2.19t \).
To find the roots we set x(t) = 0, as x(t) = f(t)g(t),
we have f(t) = 0 or g(t) = 0.
- For g(t) = 0
\[
\begin{gather}
g(t)=0.20\cos2.19t+0.01\sin 2.19t=0\\[5pt]
0.01\sin 2.19t=-0.20\cos2.19t\\[5pt]
\frac{\sin 2.19t}{\cos2.19t}=-{\frac{0.20}{0.01}}\\[5pt]
\tan 2.19t=-20\\[5pt]
2.19t=\arctan(-20)\\[5pt]
t=\frac{1}{2.19}\left[-\arctan(20)+n\pi\right]\\[5pt]
t=\frac{1}{2.19}\left[-1.52+n\pi \right]
\end{gather}
\]
with n = 1, 2, 3,..., for these values of t, we have the roots of the function
g(t), the first four values will be, for n = 1, 2, 3, and 4, respectively,
t = 0.74, 2.17, 3.60 and 5.04 (Graph 1).

- For f(t) = 0
\[
\begin{gather}
f(t)=\operatorname{e}^{-0.12t}=0\\[5pt]
\operatorname{e}^{-t}=0
\end{gather}
\]
as there is no t that satisfies this equality, the function f(t) does not intersect
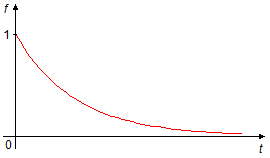
the t-axis.For any real value of t the function will always be positive, f(t) > 0.
Differentiation of the expression f(t)
\[
\begin{gather}
\frac{df}{dt}=-0.12\operatorname{e}^{-0.12t}
\end{gather}
\]
for any real value of t, the derivative will always be negative
\( \left(\frac{df(t)}{dt}<0\right) \)
and the function always decreases. Setting
\( \frac{df(t)}{dt}=0 \)
we find the maximum and minimum points of the function.
\[
\begin{gather}
\frac{df}{dt}=-0.12\operatorname{e}^{-0.12t}=0\\[5pt]
\operatorname{e}^{-0.12t}=\frac{0}{-0.12}\\[5pt]
\operatorname{e}^{-0.12t}=0
\end{gather}
\]
as there is no t that satisfies this equality, there are no maximum or minimum points of the
function.The second derivative of the expression f(t)
\[
\begin{gather}
\frac{d^{2}f}{dt^{2}}=-0.12(-0.12)\operatorname{e}^{-0.12t}\\[5pt]
\frac{d^{2}f}{dt^{2}}=0.01\operatorname{e}^{-0.12t}
\end{gather}
\]
for any value of real t, the second derivative will always be positive
\( \left(\frac{d^{2}f(t)}{dt^{2}}>0\right) \)
and the function has a concave upwards. Setting
\( \frac{d^{2}f(t)}{dt^{2}}=0 \)
we find inflection points in the function.
\[
\begin{gather}
\frac{d^{2}f}{dt^{2}}=0.01\operatorname{e}^{-0.12t}=0\\[5pt]
\operatorname{e}^{-0.12t}=\frac{0}{0.01}\\[5pt]
\operatorname{e}^{-0.12t}=0
\end{gather}
\]
as there is no t that satisfies this equality, there are no inflection points in the function.For t = 0 the expression of f(0)
\[
\begin{gather}
f(0)=\operatorname{e}^{-0.12.0}\\[5pt]
f(0)=\operatorname{e}^{-0}\\[5pt]
f(0)=1
\end{gather}
\]
As the variable t represents time, we do not calculate negative values, t<0, for t
tending to infinity
\[
\begin{gather}
\lim_{t\rightarrow \infty }f(t)=\lim_{t\rightarrow \infty}\operatorname{e}^{-0.12t}=\lim_{t\rightarrow \infty}{\frac{1}{\operatorname{e}^{0,12t}}}=0
\end{gather}
\]
From the above analysis, we plotted the graph of f versus t (Graph 2).
As x(t) = f(t)g(t), the combination of graphs produces a curve that oscillates like the cosine function damped by the exponential (Graph 3).
advertisement

Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .