Solved Problem on One-dimensional Motion
advertisement   



A cyclist A starts a race from the rest, accelerating 0.50 m/s2. At this moment, another cyclist B passes him, with a constant speed of 5.0 m/s and in the same direction as cyclist A.
a) After how long, after the start, the cyclist A reaches the cyclist B?
b) What is the speed of cyclist A when reaching cyclist B?


Problem data:
  • Initial speed of cyclist A:    v0A = 0;
  • Acceleration of cyclist A:    aA = 0.50 m/s2;
  • Speed of cyclist B:    vB = 5.0 m/s.
Problem diagram:

Figure 1

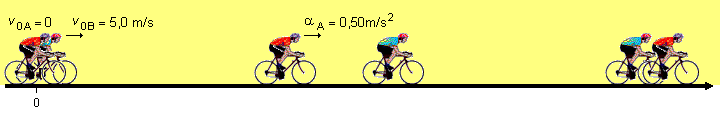
We choose a reference frame pointing to the right, with origin in the position where cyclist A begins its race (Figure 1).

Solution

Cyclist A has an acceleration, equation of displacement as a function of time with constant acceleration is given by
\[ \bbox[#99CCFF,10px] {S=S_{0}+v_{0}t+\frac{a}{2}t^{2}} \]
for cyclist A
\[ \begin{gather} S_{A}=S_{0 A}+v_{0 A}t+\frac{a_{A}}{2}t^{2}\\ S_{A}=0+0\times t+\frac{0.50}{2}t^{2}\\ S_{A}=\frac{0.50}{2}t^{2}\\ S_{A}=0.25t^{2} \tag{I} \end{gather} \]
The equation of velocity as a function of time is given by
\[ \bbox[#99CCFF,10px] {v=v_{0}+at} \]
for cyclist A
\[ \begin{gather} v_{A}=v_{0 A}+a_{A}t\\ v_{A}=0+0.50t\\ v_{A}=0.50t \tag{II} \end{gather} \]
Cyclist B has a constant speed, equation of displacement as a function of time with constant speed is given by
\[ \bbox[#99CCFF,10px] {S=S_{0}+vt} \]
for cyclist B
\[ \begin{gather} S_{B}=S_{0 B}+v_{B}t\\ S_{B}=0+5.0 t\\ S_{B}=5.0 t \tag{III} \end{gather} \]
Expressions (I) and (II) are the functions representing the position of the cyclists.

a) When cyclist A reaches cyclist B, both have the same position in the trajectory we can equate expressions (I) and (III) imposing the condition
\[ \begin{gather} S_{A}=S_{B}\\ 0.25t^{2}=5.0t\\ 0.25t^{2}-5.0t=0 \end{gather} \]
factoring 0.25t
\[ 0.25t(t-20.0)=0 \]
solving this equation, we have two cases, \( 0.25t=0 \) or \( t-20.0=0 \), in the first case, we have
\[ \begin{gather} 0.25t=0\\ t=\frac{0}{0.25}\\ t=0 \end{gather} \]
and for the second case if we do
\[ \begin{gather} t-20.0=0\\ t=20.0\;\text{s} \end{gather} \]
The value t = 0 represents the instant where cyclist B is passing through cyclist A, which is coming out of the rest and accelerating, which is the first meet when we started counting time. The value t = 20.0 s represents the time interval that the cyclist A increases its speed and overtaking cyclist B, this is the instant of the overtaking we are looking for.
Cyclist A takes 20,0 s to reach cyclist B.

b) Substituting the value found in the previous item in the expression (II), we find the speed of A on overtaking
\[ v_{A}=0.50\times 20.0 \]
\[ \bbox[#FFCCCC,10px] {v_{A}=10.0\;\text{m/s}} \]
advertisement   

Creative Commons License
Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .