Solved Problem on Contour Integration

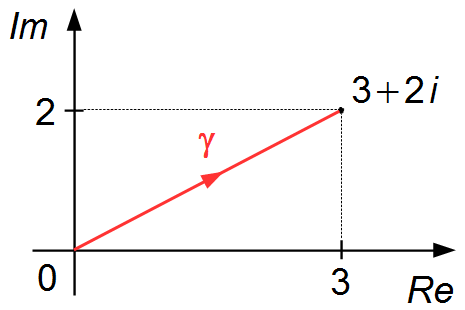
g) \( f(z)=x^{2}-y^{2}+i(x-y^{2}) \), along the path from the origin to point 3+2i.

Parameterization of the γ curve (Figure 1)

The parameterization of a line is given by

\[ \begin{gather} \bbox[#99CCFF,10px] {z(t)=z_{1}+t(z_{2}-z_{1})} \end{gather} \]

where z₁ and z₂ are the start and end points of the curve, \( z_{1}=0+0i \) and \( z_{2}=3+2 i \)

\[ \begin{gather} z(t)=(0+0i)+t[(3+2 i)-(0+0i)]\\[5pt] \qquad \qquad \qquad \qquad z(t)=3 t+2 it\qquad ,\qquad 0\leqslant t\leqslant 1 \tag{I} \end{gather} \]

Figure 1

Derivative of z(t) = t+it

\[ \begin{gather} z'(t)=\frac{dz}{dt}=3+2i \tag{II} \end{gather} \]

The contour integral is given by

\[ \begin{gather} \bbox[#99CCFF,10px] {\int f(z)\;dz=\int f(z(t))z'(t)\;dt} \tag{III} \end{gather} \]

Integration of \( f(z)=x^{2}-y^{2}+i(x-y^{2}) \)

\[ \begin{gather} f(z)=x^{2}-y^{2}+i(x-y^{2}) \tag{IV} \end{gather} \]

using expression (I) we have f(z(t))

\[ \begin{gather} z(t)=\underbrace{\ 3t\ }_{x(t)}+\underbrace{\ 2t\ }_{y(t)}i \tag{V} \end{gather} \]

substituting the values of x(t) and y(t) of expression (V) into expression (IV)

\[ \begin{gather} f(z(t))=(3t)^{2}-(2t)^{2}+i\left[(3t)-(2t)^{2}\right]\\ f(z(t))=9t^{2}-4t^{2}+i\left(3t-4t^{2}\right) \tag{VI} \end{gather} \]

substituting expressions (II) and (VI) into expression (III)

\[ \begin{split} \int f(z)\;dz &=\int_{0}^{1}\left[9t^{2}-4t^{2}+i\left(3t-4t^{2}\right)\right](3+2i)\;dt=\\[5pt] &=\int_{0}^{1}\left(5t^{2}+3it-4it^{2}\right)(3+2i)\;dt=\\[5pt] &=\int_{0}^{1}\left(15t^{2}+9it-12it^{2}+10it^{2}+6i^{2}t-8i^{2}t^{2}\right)\;dt=\\[5pt] &=\int_{0}^{1}\left(15t^{2}+9it-2it^{2}+6\times(-1)t-8\times(-1)t^{2}\right)\;dt=\\[5pt] &=\int_{0}^{1}\left(15t^{2}+9it-2it^{2}-6t+8t^{2}\right)\;dt=\\[5pt] &=\int_{0}^{1}\left(23t^{2}+9it-2it^{2}-6t\right)\;dt=\\[5pt] &=23\times\left(\left.\frac{t^{3}}{3}\right|_{0}^{1}\right)+9i\times\left(\left.\frac{t^{2}}{2}\right|_{0}^{1}\right)-2i\times\left(\left.\frac{t^{3}}{3}\right|_{0}^{1}\right)-6\times\left(\left.\frac{t^{2}}{2}\right|_{0}^{1}\right)=\\[5pt] &=23\times\left(\frac{1}{3}\right)+9i\times\left(\frac{1}{2}\right)-2i\times\left(\frac{1}{3}\right)-6\times\left(\frac{1}{2}\right)=\\[5pt] &=\frac{23}{3}+\frac{9}{2}i-\frac{2}{3}i-\frac{6}{2}=\frac{46+27i-4i-18}{6}=\frac{28+23i}{6} \end{split} \]

\[ \begin{gather} \bbox[#FFCCCC,10px] {\int_{0}^{3+2i}f(z)\;dz=\frac{28+23i}{6}} \end{gather} \]

Fisicaexe - Physics Solved Problems by Elcio Brandani Mondadori is licensed under a Creative Commons Attribution-NonCommercial-ShareAlike 4.0 International License .